A new analytical approach of nonlinear thermal buckling of FG-GPLRC circular plates and shallow spherical caps using the FSDT and Galerkin method
Author affiliations
DOI:
https://doi.org/10.15625/0866-7136/17932Keywords:
nonlinear stability, first-order shear deformation theory, FG-GPLRC, thermal load, Galerkin methodAbstract
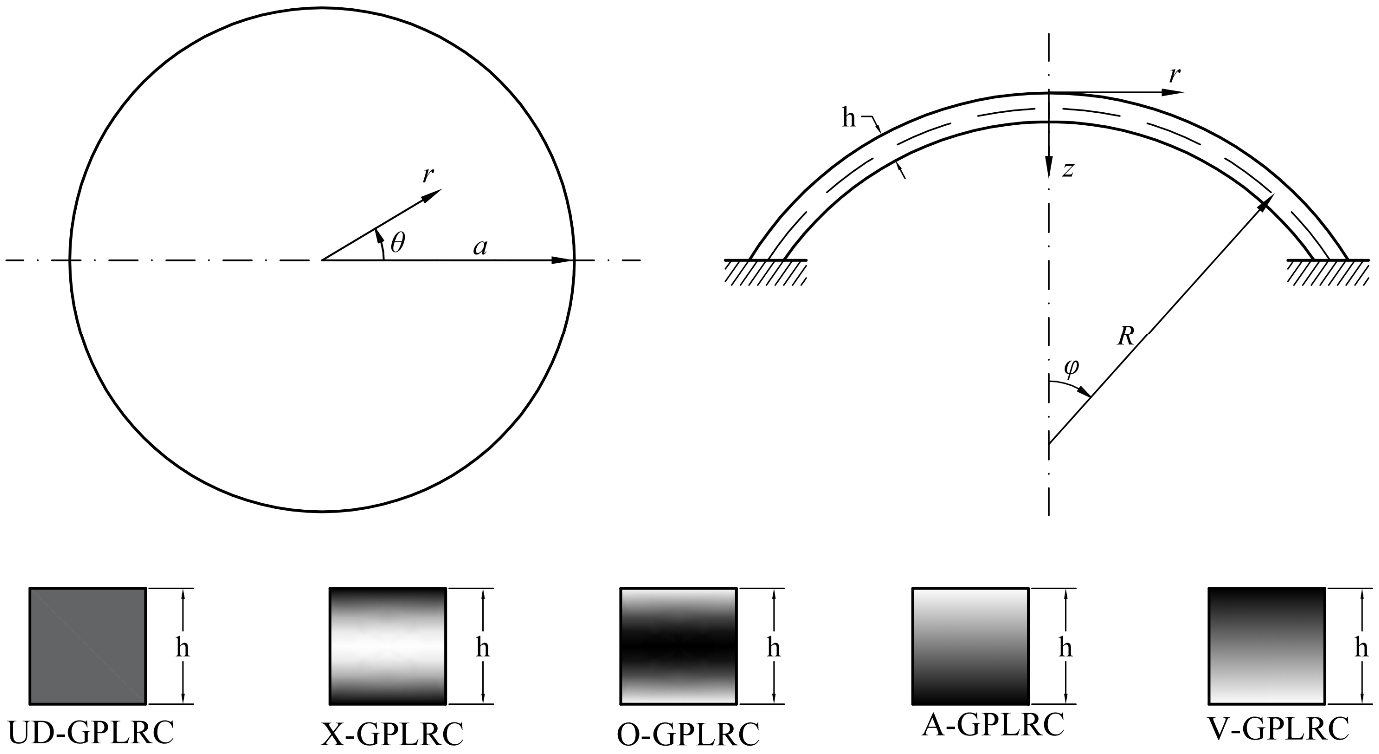
A new analytical approach for nonlinear thermal buckling of Functionally Graded Graphene Platelet Reinforced Composite (FG-GPLRC) circular plates and shallow spherical caps using the first-order shear deformation theory (FSDT) is presented in this paper. The circular plates and shallow spherical caps are assumed to be subjected to uniformly distributed thermal loads. By applying the Galerkin method, the relations between thermal load–deflection are achieved to determine the postbuckling behavior and critical buckling loads of the considered structures. Special effects on the nonlinear thermal behavior of circular plates and shallow spherical caps with five different material distribution laws, different Graphene platelet (GPL) mass fractions, and geometrical dimensions are explored and discussed in numerical examples.
Downloads
Metrics
References
M. M. Najafizadeh and B. Hedayati. Refined theory for thermoelastic stability of functionally graded circular plates. Journal of Thermal Stresses, 27, (2004), pp. 857–880. DOI: https://doi.org/10.1080/01495730490486532
L. V. Tran, C. H. Thai, and H. Nguyen-Xuan. An isogeometric finite element formulation for thermal buckling analysis of functionally graded plates. Finite Elements in Analysis and Design, 73, (2013), pp. 65–76. DOI: https://doi.org/10.1016/j.finel.2013.05.003
D. H. Bich, D. V. Dung, and V. H. Nam. Nonlinear axisymmetric dynamic buckling and vibration of functionally graded shallow spherical shells under external pressure including temperature effects resting on elastic foundation. In Vietnam National Conference on Mechanics of Deformable Solids, Ho Chi Minh City, (2013), pp. 101–110.
D. H. Bich, D. V. Dung, and V. H. Nam. Nonlinear axisymmetric dynamic analysis of shallow spherical shells with functionally graded coatings under external pressure including temperature effects and resting on elastic foundation. In Vietnam National Conference on Mechanic Engineering, Hanoi, (2014), pp. 25–30.
R. Shahsiah, M. R. Eslami, and R. Naj. Thermal instability of functionally graded shallow spherical shell. Journal of Thermal Stresses, 29, (2006), pp. 771–790. DOI: https://doi.org/10.1080/01495730600705406
N. T. Phuong, V. H. Nam, and D. T. Dong. Nonlinear vibration of functionally graded sandwich shallow spherical caps resting on elastic foundations by using first-order shear deformation theory in thermal environment. Journal of Sandwich Structures & Materials, 22, (2018), pp. 1157–1183. DOI: https://doi.org/10.1177/1099636218782645
T. Q. Minh, D. T. Dong, V. M. Duc, N. V. Tien, N. T. Phuong, and V. H. Nam. Nonlinear axisymmetric vibration of sandwich FGM shallow spherical caps with lightweight porous core. In Lecture Notes in Civil Engineering, pp. 381–389. Springer Singapore, (2021). DOI: https://doi.org/10.1007/978-981-16-7160-9_38
J. Huo, G. Zhang, A. Ghabussi, and M. Habibi. Bending analysis of FG-GPLRC axisymmetric circular/annular sector plates by considering elastic foundation and horizontal friction force using 3D-poroelasticity theory. Composite Structures, 276, (2021). DOI: https://doi.org/10.1016/j.compstruct.2021.114438
D. Liu, Y. Zhou, and J. Zhu. On the free vibration and bending analysis of functionally graded nanocomposite spherical shells reinforced with graphene nanoplatelets: Three-dimensional elasticity solutions. Engineering Structures, 226, (2021). DOI: https://doi.org/10.1016/j.engstruct.2020.111376
Y. Wang, R. Zeng, and M. Safarpour. Vibration analysis of FG-GPLRC annular plate in a thermal environment. Mechanics Based Design of Structures and Machines, 50, (2020), pp. 352–370. DOI: https://doi.org/10.1080/15397734.2020.1719508
Downloads
Published
How to Cite
License
This work is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.
