Vibration analysis of Timoshenko microbeams made of functionally graded materials on a Winkler-Pasternak elastic foundation
Author affiliations
DOI:
https://doi.org/10.15625/0866-7136/20579Keywords:
FGM, microbeam, nondimensional frequency, MCSTAbstract
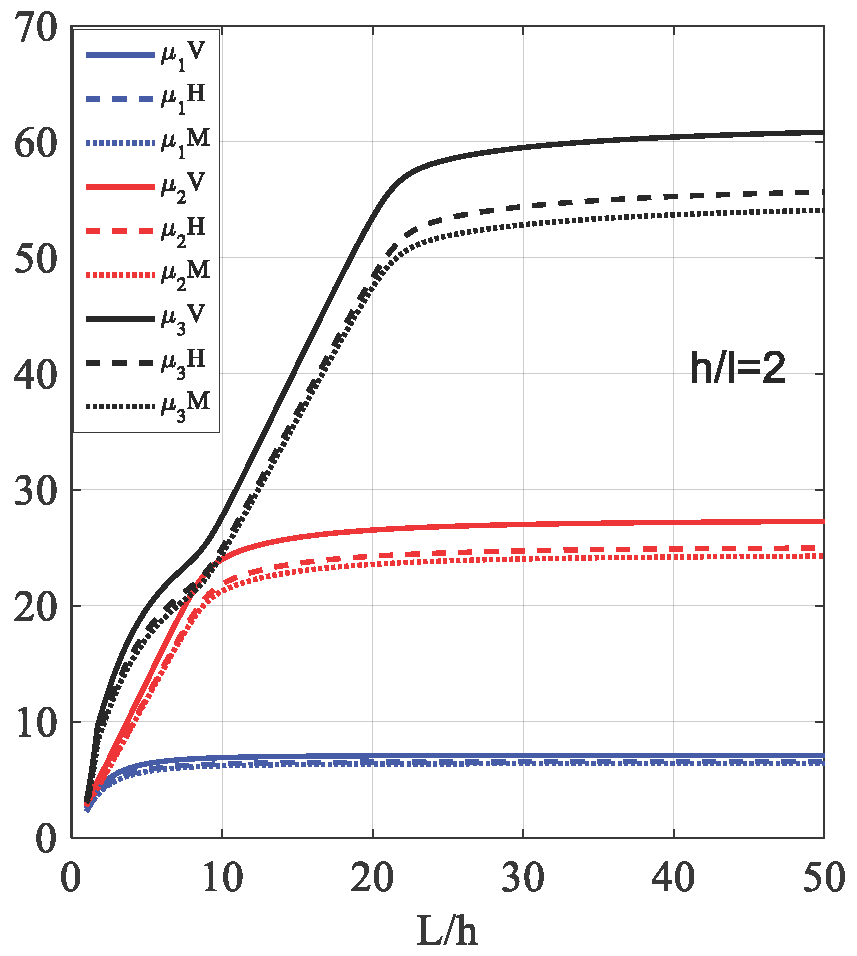
In this work, the free vibration analysis of Timoshenko microbeams made of the Functionally Graded Material (FGM) on the Winkler-Paternak elastic foundation based on the Modified Coupled Stress Theory (MCST) is investigated. Material characteristics of the beam vary throughout the thickness according to the power distribution and are estimated through Mori–Tanaka, Hashin-Shtrikman and Voigt homogenization techniques. The Timoshenko microbeam model considering the length scale parameter is applied. The free vibration differential equations of FGM microbeams are established based on the Finite Element Method (FEM) and Kosmatka’s shape functions. The influences of the size-effect, foundation, material, and geometry parameters on the vibration frequency are then analyzed. It is shown that the study can be applied to other FGMs as well as more complex beam structures.
Downloads
References
F. Yang, A. C. M. Chong, D. C. C. Lam, and P. Tong. Couple stress based strain gradient theory for elasticity. International Journal of Solids and Structures, 39, (2002), pp. 2731–2743.
D. C. C. Lam, F. Yang, A. C. M. Chong, J. Wang, and P. Tong. Experiments and theory in strain gradient elasticity. Journal of the Mechanics and Physics of Solids, 51, (2003), pp. 1477–1508.
M. Şimşek and J. N. Reddy. A unified higher order beam theory for buckling of a functionally graded microbeam embedded in elastic medium using modified couple stress theory. Composite Structures, 101, (2013), pp. 47–58.
M. Şimşek and J. N. Reddy. Bending and vibration of functionally graded microbeams using a new higher order beam theory and the modified couple stress theory. International Journal of Engineering Science, 64, (2013), pp. 37–53.
R. Ansari, R. Gholami, and S. Sahmani. Free vibration analysis of size-dependent functionally graded microbeams based on the strain gradient Timoshenko beam theory. Composite Structures, 94, (2011), pp. 221–228.
M. H. Kahrobaiyan, M. Asghari, and M. T. Ahmadian. A Timoshenko beam element based on the modified couple stress theory. International Journal of Mechanical Sciences, 79, (2014), pp. 75–83.
L.-L. Ke and Y.-S. Wang. Size effect on dynamic stability of functionally graded microbeams based on a modified couple stress theory. Composite Structures, 93, (2011), pp. 342–350.
H.-T. Thai, T. P. Vo, T.-K. Nguyen, and J. Lee. Size-dependent behavior of functionally graded sandwich microbeams based on the modified couple stress theory. Composite Structures, 123, (2015), pp. 337–349.
M. Salamat-talab, A. Nateghi, and J. Torabi. Static and dynamic analysis of third-order shear deformation FG micro beam based on modified couple stress theory. International Journal of Mechanical Sciences, 57, (2012), pp. 63–73.
B. Akgöz and Ö. Civalek. Free vibration analysis of axially functionally graded tapered Bernoulli–Euler microbeams based on the modified couple stress theory. Composite Structures, 98, (2013), pp. 314–322.
X. Chen, X. Zhang, Y. Lu, and Y. Li. Static and dynamic analysis of the postbuckling of bi-directional functionally graded material microbeams. International Journal of Mechanical Sciences, 151, (2019), pp. 424–443.
N. Shafiei, A. Mousavi, and M. Ghadiri. On size-dependent nonlinear vibration of porous and imperfect functionally graded tapered microbeams. International Journal of Engineering Science, 106, (2016), pp. 42–56.
M. A. R. Loja, J. I. Barbosa, and C. M. Mota Soares. A study on the modeling of sandwich functionally graded particulate composites. Composite Structures, 94, (2012), pp. 2209–2217.
J. B. Kosmatka. An improved two-node finite element for stability and natural frequencies of axial-loaded Timoshenko beams. Computers & Structures, 57, (1995), pp. 141–149.
Downloads
Published
How to Cite
License

This work is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.




